Solstick: How it works
To measure the size of the Earth, we can use the fact that shadows cast by the Sun are different lengths in different places at the same time. If the Earth were flat, all the shadows would be the same everywhere at a particular time, but because it is curved like a ball, some shadows are longer than others.
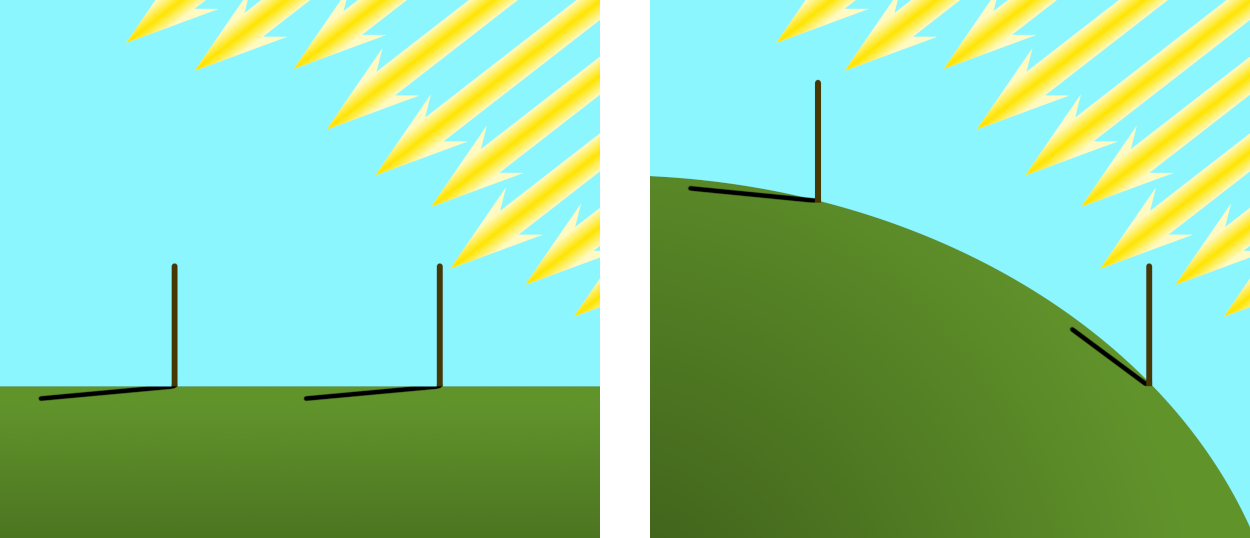
So, to estimate the size of the "ball" you just need to measure the size and direction of the shadows at two difference places at the same time. Then, when you have measured the distance between the two places, you can use the angles to work out how big the entire globe is.
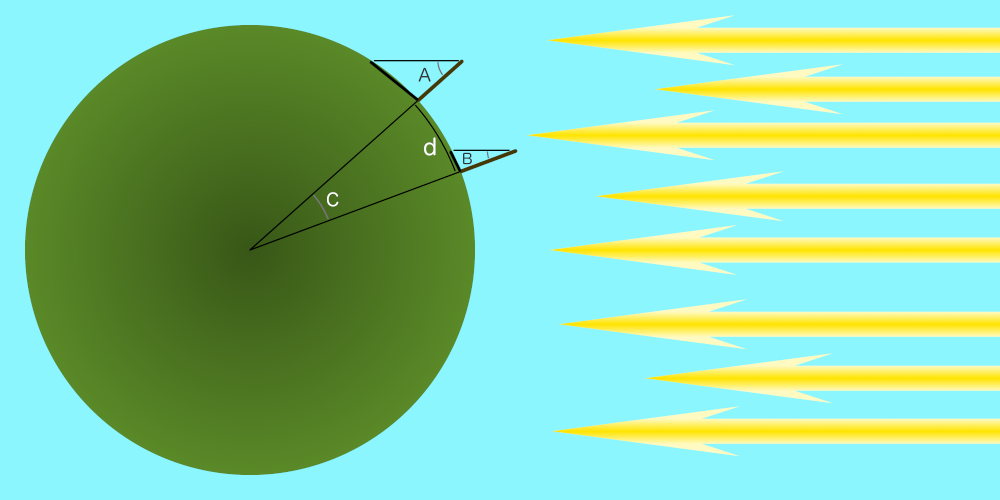
With telephones and accurate clocks, it is quite easy to get two measurements in different places at the same time, but thousands of years ago when there were no telephones or radios, no accurate clocks, and not even totally reliable calendars, it was almost impossible, so early scientists had to think up a cleverer way. One of the first to do this was Eratosthenes who lived in north Africa more than two thousand years ago. We don't know exactly how he did it, but it was probably something like the method described by a astronomer called Cleomedes hundreds of years after Eratosthenes had died.
Getting the size from one shadow
The problem and its solution come in two parts: How do you get two measurements a long way apart at the same time of day? And how do you get them on the same day?
The first part is quite easy. When the Sun rises in the morning, the shadows are really long as the Sun is low in the sky. As the Earth spins, the Sun appears to rise and the shadows get shorter until the middle of the day, and then start getting longer again through the afternoon and towards evening. So, if you measure the shadow when it is shortest on that day, it must be midday wherever you are!
Getting the same day is a bit trickier, but here there is a clever solution that uses the seasons. The spin of the Earth (that gives us day and night) is tilted a bit compared to its orbit around the Sun (which gives us years). When the half of the Earth you are in (north or south) is tilted towards the Sun you get summer, when it is tilted away you get winter.
Because of this, during the winter the Sun never gets as high in the sky as during the summer, and so the shadows are longer. However, as you go through spring and into summer, the shadows get shorter until on one particular day - the summer Solstice - the midday shadow is the shortest of the year. After that the shadow gets longer again as you go towards autumn and back to winter.
Therefore, if the early scientists measured the midday shadow every day in the summer and found the day when it was shortest, they would know it was the summer solstice, no matter where they were.
To make this even easier, there was a town to the south of Eratosthenes called Syene (now Aswan) which it was thought had no shadows at all at midday on the summer solstice as the Sun was directly overhead. This isn't quite right, but it is close enough, so all Eratosthenes had to do was measure the midday shadow on the solstice where he was due north of Syene, and then somehow find the distance from there to Syene (possibly by sending a servant on the long journey to measure it!)
Nowadays, we know what day the solstice is and when the middle of the day is (it is 1PM not 12 since we are on British Summer Time - you may remember the clocks going forward in March). And using your postcode, we can work out where you are and so how far north of Syene you are.
So, that is how you will be following in the footsteps of ancient scientists and using your own measurements to estimate the size of the Earth.
Getting the size from two shadows

Of course, in order to work out the result using just one shadow, we need to know the distance from where your stick was to the Tropic of Cancer (an imaginary line which runs round the world a bit north of the Equator). To work that out, we need a map showing quite a lot of the Earth, which is actually quite hard to make without already knowing the size of the Earth, so this is perhaps a bit of a cheat!
However, if we get a lot of results from you all, we can miss out that step by using pairs of shadows sticks. By using each pair of results, we can estimate of the size of the Earth without knowing the distance to the Tropic of Cancer - we just need to know how far apart the sticks were, which we can find using a much smaller map just of the UK!
Remember to check back after the experiment is finished to see the final results using all the pairs of measurements

